In one of
Floyd Sweet's papers, Magnetic Resonance by Floyd A. Sweet .PH. D, Floyd mentions,
Paul Raymond Jensen three times:
Referring to the Jensen machine stated:
natural magnetic resonance freq = 2.80GHz the nuclear magnetic resonance of a free
electron when charges in magnetic states are induced by magnetic field the changes in
states causes a condition called electron paramagnetic resonance, or EPR. The EPR of a
free electron is 2.80 H MC. Where H is in gauss. This should be the initial state of
the defining mathematical format.
and:
Resonance frequencies may be
maintained quite constant at high power levels so long as the load remains constant.
We are all familiar with AM and FM propagation, where in the case as AM, the voltage
amplitude varies, and with FM, the frequency is modulated.
However, the output
power sees a constant load impedance, that of the matched antenna system. If this
changes, the input to the antenna is mismatched, and standing waves are generated
resulting in a loss of power. The frequency is a forced response and remains constant.
Power is lost and efficiency becomes less and less, depending on the degree of
mismatch. Let’s assume the Jensen amplifying transformer is in a resonating condition.
Its output is connected to a transmission line which is X number of miles long.
Without any customer load at all, power will be required to change the line. This will
present capacitive reactance, XC = 1/2 fc.
and:
The power factor cos ?
angle will be leading, though negligible on short systems. The effect must be reckoned
with on multiple grid long systems operating above 60 KV. What we have is a capacitor
and the effects are evident as line impedance. Another parameter is varying power
factor due to changing inductive loads.Taken together this forms a complex impedance
load continually varying and this is what the “Jensen” machine will “see” when
connected to power distributing network grids. Such a resonant machine will never
sustain resonance as shown in the sketch. The circuit consists of a capacitor in
series with an inductor and this is a series resonant circuit of minimum impedance and
maximum current. Theoretically, the current limiting is effected by series resistance
in the circuit including the resistance of the inductor,
A paper was released
also:
A Free-Energy Device by Paul Raymond Jensen
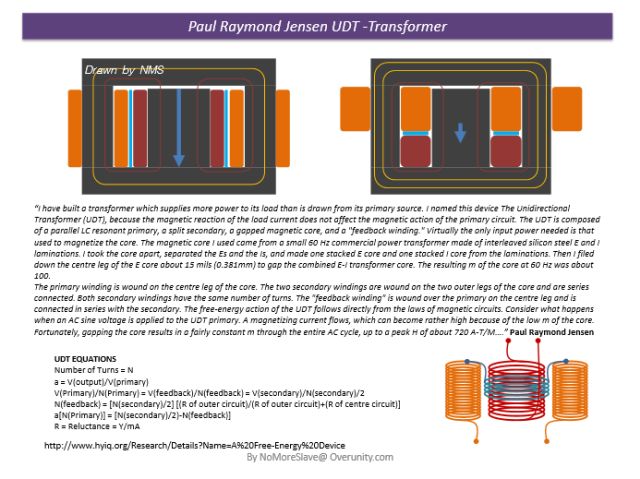
I have built a
transformer which supplies more power to its load than is drawn from its primary
source. I named this device The Unidirectional Transformer (UDT), because the magnetic
reaction of the load current does not affect the magnetic action of the primary
circuit. The UDT is composed of a parallel LC resonant primary, a split secondary, a
gapped magnetic core, and a "feedback winding." Virtually the only input power needed
is that used to magnetize the core. The magnetic core I used came from a small 60 Hz
commercial power transformer made of interleaved silicon steel E and I laminations. I
took the core apart, separated the Es and the Is, and made one stacked E core and one
stacked I core from the laminations. Then I filed down the centre leg of the E core
about 15 mils (0.381mm) to gap the combined E-I transformer core. The resulting m of
the core at 60 Hz was about 100.
The primary winding is wound on the centre leg
of the core. The two secondary windings are wound on the two outer legs of the core
and are series connected. Both secondary windings have the same number of turns. The
"feedback winding" is wound over the primary on the centre leg and is connected in
series with the secondary. The free-energy action of the UDT follows directly from the
laws of magnetic circuits. Consider what happens when an AC sine voltage is applied to
the UDT primary. A magnetizing current flows, which can become rather high because of
the low m of the core. Fortunately, gapping the core results in a fairly constant m
through the entire AC cycle, up to a peak H of about 720 A-T/M.
This results in
a constant primary inductance, which permits parallel LC resonation. Resonating the
primary reduces the magnetizing power to that necessary to match I2* R losses in the
primary and the hysteresis losses in the core. Magnetizing the core results in an AC
sine voltage being induced across the secondary. The magnetic coupling between the
primary and the secondary is very high, but the core area within each secondary
winding is only one-half that of the primary. This means that the volts/turn of the
secondary will be only one-half that of the primary. For the secondary voltage to
equal the primary voltage, the secondary must have two times the number of turns in
the primary.
The primary also induces a voltage across the feedback coil, but
the purpose and characteristics of the feedback coil will be explained later. When a
current is drawn from the output, the two secondary windings each generate a
magnetomotive force (MMF) directed against the MMF of the primary. The MMF of each
secondary winding "sees" a series-parallel magnetic circuit through the transformer
core. One magnetic circuit, "seen" by each secondary winding, is through the centre
leg of the core. The other magnetic circuit "seen" by each secondary winding is
through the two outer legs of the core. The resulting magnetic flux generated by the
MMFs of the two secondary windings is dependent upon the reluctances of each of the
magnetic circuits.
Because the centre leg is gapped, it has a higher reluctance
than do the outer legs. This means that less magnetic flux from the secondary will
pass through the centre leg than will pass through the outer legs.
In my
transformer, the reluctances of the magnetic circuits through the centre leg were
three times higher than the reluctances of the magnetic circuits through both outer
legs. This was difficult to achieve and required hours of filing, polishing and
fitting of the E and I cores. The alternative was to increase the gap, which was not
acceptable in my particular design because I was driving the transformer at 60 Hz and
could not afford any additional loss of m in the core.
Since the reluctances of
the "centre leg circuits" were three times higher than the reluctances of the "outer
leg circuits," one-quarter of the secondary flux passed through the centre leg, while
three-quarters of the secondary flux passed through both outer legs. The magnetic flux
from the two secondary windings cancels in the "outer leg circuits," leaving only
one-quarter of the total flux generated by the output current to react back upon the
primary. This resulted in a current gain in the secondary, relative to the primary.
Lenz's law was bypassed, and free-energy resulted. An alternate explanation for the
current gain in the UDT is to consider each secondary winding as acting as the primary
winding for the other secondary winding when an output current is drawn because the
two secondary windings generate geometrically opposing fields.
Now consider the
"feedback winding." It is connected in series with the secondary and is wound over the
primary winding on the centre leg of the core. When the core is magnetized, an induced
voltage will appear across the feedback winding which will subtract from the voltage
across the secondary. The purpose of the feedback winding is to cancel the remaining
secondary flux passing through the centre leg of the core. It effectively isolates the
currents in the primary and the secondary at the cost of a reduced output voltage. The
feedback winding generates a magnetic flux equal and opposite to the residual magnetic
flux from the secondary when an output current is drawn.
Given the above
example, where three-quarters of the secondary flux self-cancels in the "outer leg
circuits," the feedback coil will only have to oppose one-quarter of the total
secondary flux. Since the feedback winding has two times the core area of the
secondary windings and carries the full output current, it need have only one-quarter
the number of turns of each secondary winding. However, this will reduce the output
voltage by 25 percent. Therefore, to achieve the originally desired output voltage,
the total number of secondary turns must be increased by the factor 4/3; the feedback
coil must then have one-quarter of the number of turns of each secondary winding in
this new secondary circuit.
Given the condition in which the feedback coil
perfectly cancels all the residual secondary flux through the centre leg of the core,
the power drawn from the output will be nearly independent of the primary input power.
The primary input will be the magnetizing power and nothing more. The output power
will have a negligible phase angle (due to the leakage inductance) if the m of the
core (as seen by the primary) is at least 100. In practice, it is best if the feedback
winding is short a turn or two, thereby preventing series inductance in the output at
the cost of a small increase in the primary input power. A parallel resonant primary
circuit allows for great input power reduction while ensuring voltage stability and
linear operation under varying output loads.
The UDT can be used without a
resonant primary circuit for the amplification of any time-varying signal. The main
flaws of the UDT are the (normally) low primary m and the very long secondary wire
required to ensure isolation of the input from the output. A single or double stack of
E-I laminations seems to provide the optimum core geometry, all factors considered. At
high frequencies it becomes practical to use ferrite cores with "centre leg circuit"
reluctances less than their "outer leg circuit" reluctances because the volts/turn of
each winding can be made very high. Conventional transformer design techniques should
be used once the basic UDT topology has been determined.
I have invented and
developed the UDT on my own, without benefit of any knowledge of other free-energy
devices, if they exist, which utilize the basic principles of UDT operation. Please
feel free to use this information as you desire. However, I hope that no one will
attempt to patent and control this type of transformer. The time on Planet Earth is 15
minutes before midnight; there is no time left to waste.
Free-energy technology
is not meant to be controlled by vain and greedy parasites who wish to use a gift from
God to exploit their fellow man. Free-energy technology represents a spiritual
transition of the human race. Free-energy is not meant to be owned, period!
UDT EQUATIONS
Number of Turns = N
a = V(output)/V(primary)
V(Primary)/N(Primary) = V(feedback)/N(feedback) = V(secondary)/N(secondary)/2
N(feedback) = [N(secondary)/2] [(R of outer circuit)/(R of outer circuit)+(R of centre
circuit)]
a[N(Primary)] = [N(secondary)/2)-N(feedback)]
R = Reluctance =
Y/mA
back to linkpage
suggestion
read and sign my guestbook

